M347
Mathematical Statistics
You will enjoy M347 if…
- you’re interested in why stats works mathematically
- you love the idea of taking a deep dive into Bayes
- you’re curious about when your data science models are valid (and when they aren’t!)
- you aren’t afraid of a little algebra 😁
Coursebooks
Four coursebooks, four extra exercise books and a handbook! (click to enlarge)
Interactive web-based content
M347 units were written to be browser-friendly (bonus to hard-copy and pdf versions)…
. . .
Screencasts (short lectures) pre-recorded for many key topics.
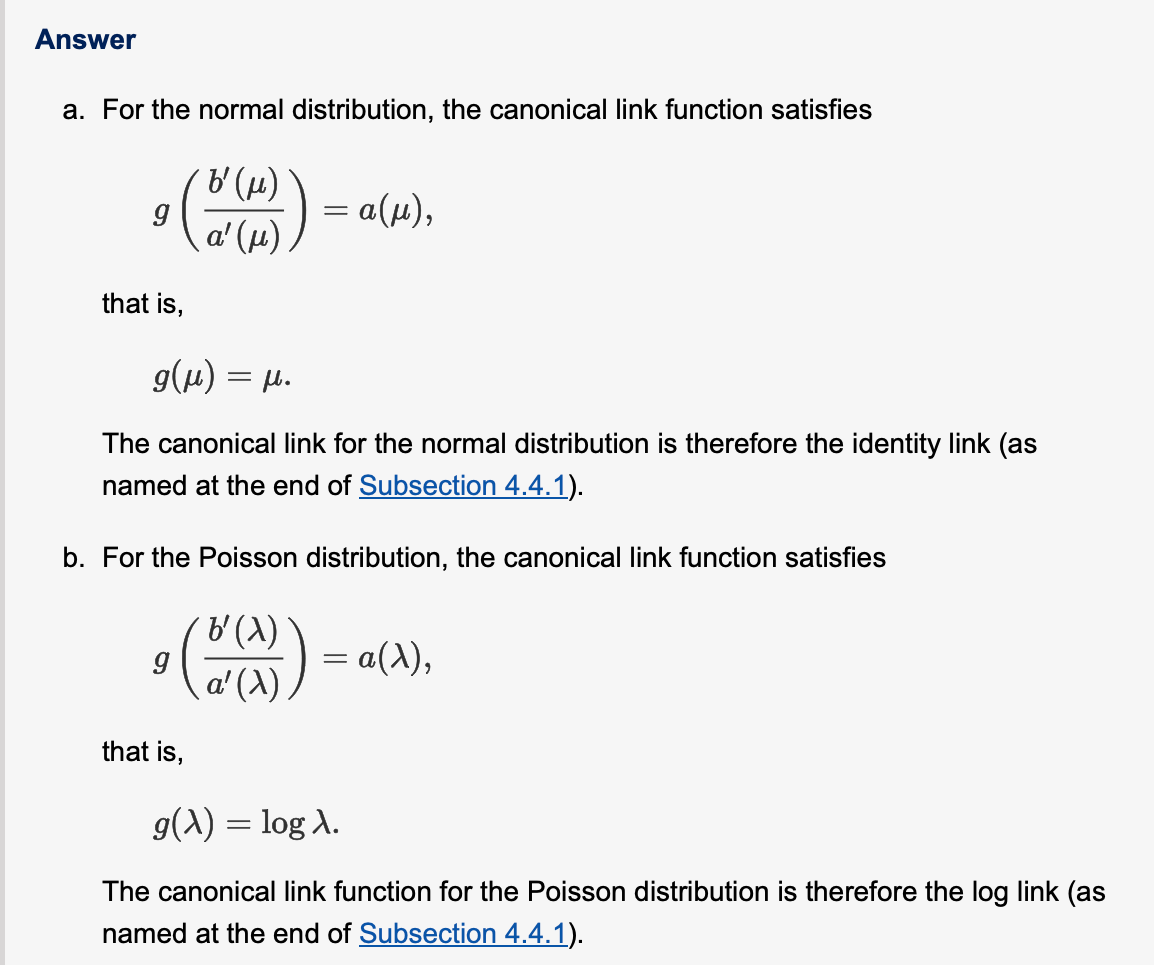
The standard bivariate normal distribution:
\[f(z,w) = \frac{1}{2\pi \sqrt{1-\rho^2}}\exp \left[-\frac{1}{2} \left(\frac{z^2-2\rho zw + w^2}{1-\rho^2}\right)\right]\]
. . .
So if the correlation \(\rho = 0\), this simplifies to: \[f(z,w) = \frac{1}{2\pi} \exp \left[-\frac{1}{2} \left({z^2 + w^2}\right)\right] \]
. . .
\[f(z,w) = \frac{1}{\sqrt{2\pi}}\exp \left[-\frac{1}{2}z^2\right]\frac{1}{\sqrt{2\pi}}\exp \left[-\frac{1}{2}w^2\right] = f(z)f(w)\]
. . .
What famous result does this help us understand?
Day-in-the-life of M347
For musicians - scales; For mathematicians - exercises!
Thanks for visiting!
I enjoyed M347 as an OU student, and hope you find it as intriguing and rewarding as I did!
. . .